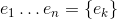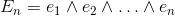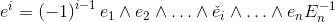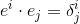Attention
This page has been tested and developed with the Mozilla browser. This page requires JavaScript in your browser. Without JavaScript or JavaScript beeing disabled, the display of this side is incomplete.
Prolog
...Though several people might be credited with conceiving the idea of "directed number", Hermann Grassmann, in his book of 1844,
developed the idea with precision and completeness that far surpassed the work of anyone else at the time...
David Hestenes
..Grassmann looked for rules for combining vectors which would fully describe the geometrical properties of directed line segments.
He noticed that two directed line segments connected end to end determined a third, which may be regarded as their sum...
David Hestenes
Den ersten Anstoss gab mir die Betrachtung des Negativen in der Geometrie;
ich gewöhnte mich, die Strecken AB und BA als entgegengesetzte Größen aufzufassen;
Hermann Grassmann - Die Ausdehnungslehre - Vorrede.
The first impulse was the consideration of the negative in geometry; I got the habit to view the distances AB and BA as opposite values;
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
distance AB = - distance BA
...woraus dann hervorging, dass, wenn A,B,C Punkte einer geraden Line sind,
dann auch allemal AB + BC = AC sei, sowohl wenn AB und BC
gleichbezeichnet sind...
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
...from which it then emerged that if A, B, C are points of a straight line,
then also always AB + BC = AC, both if AB and BC
are labeled the same...
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
...als auch wenn entgegengesetzt bezeichnet, d.h. wenn C zwischen A und B liegt.
In dem letzteren Falle waren nun AB und BC nicht als blosse Längen aufgefasst,
sondern an ihnen zugleich ihre Richtung festgehalten, vermöge deren sie eben einander engegengesetzt waren.
So drängt sich der Unterschied auf zwischen der Summe der Längen und zwischen der Summe solcher Strecken,
in denen zugleich eine Richtung festgehalten war.
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
...as well as when labeled opposite, that means C is between A and B.
In the latter case, AB and BC were not taken to be pure lengths,
but also considering their direction, which is reverse to one another.
The difference between the sum of the lengths and the sum of those distances in which the direction was considered becomes obvious.
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
Hieraus ergab sich die Forderung, den letzten Begriff der Summe nicht bloss für den Fall,
dass die Strecken gleich- oder entgegengesetzt-gerichtet waren, sondern auch für jeden anderen Fall festzustellen.
Dies kann aufs einfachste geschehen in dem das Gesetz AB + BC = AC sei, auch dann noch festgehalten wurde,
wenn A,B,C nicht in einer geraden Linie waren.
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
This resulted in the requirement to use the last concept of a sum, not only for the case when the routes were directed in the same direction or opposite,
but also for every other case.
This can be done in the simplest way when the law AB + BC = AC is also applied, when A, B, C are not in a straight line.
Hermann Grassmann - Die Ausdehnungslehre - Vorrede
References
This page is based on the concepts developed on my geometric algebra page and on my linear algebra page.
